Cyanuric acid (CYA) is a useful tool for stabilizing chlorine residuals and reducing chlorine degradation from UV radiation in sunlight. The greatest improvement in chlorine retention is seen with the addition of the first 5 ppm of CYA. Diminishing returns in UV stabilization are seen as CYA concentration increases – particularly at CYA concentrations between 20 ppm and 100 ppm where incremental increases in CYA show little incremental improvement in stabilizing chlorine residuals.
While CYA is a useful chemistry for reducing chlorine degradation from UV radiation in sunlight, the CYA interaction with the chlorine residual slows the disinfection rate of chlorine, decreases ORP, and inhibits the activity of chlorine against algae. Because of this, the use of CYA should be limited.
For indoor pools, degradation of chlorine from UV radiation in sunlight is minimal, and thus, CYA should not be used.
The effect of CYA on disinfection rates has been studied for decades. The attached reference list gives a summary of the various studies that have been conducted over the years with various pathogens including bacteria, viruses, amoeba and protozoa such as Cryptosporidium. In all of the kill rate studies published in peer reviewed scientific journals within the pool pH range of 7.2-7.8, the time it takes free chlorine to kill an organism with cyanuric acid has always been shown to be longer than without cyanuric acid.
The difficulty is in setting a limit on CYA and defining how much is too much. Since each pathogen is unique in its infective dose, chlorine kill rate and other factors, it is difficult to use a ‘one-size-fits-all’ criteria that may be applied to all pathogens and in all pools.
The following two approaches may be used to help define reasonable limits:
The Centers for Disease Control and Prevention (CDC) has conducted studies looking at the effect of CYA on Cryptosporidium (crypto) kill ratesi. The CDC was unable to achieve a 3-log reduction (99.9%) with CYA concentrations above 16 ppm. Therefore, the CDC recommends that for remediation of a diarrheal fecal accident with chlorine, the CYA concentration should be below 15 ppmii.
For normal operations then, the CYA maximum could be set at 15 ppm so that the water will not need to be drained before responding to a diarrheal fecal accident.
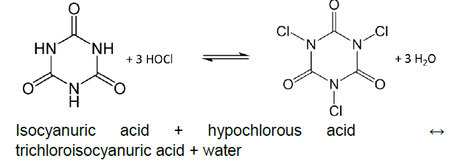
All pool chlorine sanitizers form hypochlorous acid (HOCl) when they are dissolved in water. As stated in White’s Handbook of Chlorination, “this species of chlorine is the most germicidal of all chlorine compounds with the possible exception of chlorine dioxide”iii. As such, most pool operator guides, such as the National Swimming Pool Foundation Pool and Spa Operator Handbookiv, will point out that HOCl is the active killing form of chlorine in water.
Using known chemical equilibrium constantsv, it is possible to calculate the HOCl concentration with increasing CYA concentrations. Data from the Environmental Protection Agency (EPA) shows that in order to kill Giardia, 16.7 times more monochloramine is needed than free chlorinevi. The calculated CYA concentration to reduce the HOCl concentration 16.7- fold can be determined to be 14 ppm CYA for 1 ppm free available chlorine (FAC). Thus to maintain the efficacy of FAC when compared to monochloramine, the CYA:FAC ratio should not exceed 14:1.
If higher levels of chlorine are maintained, then higher levels of CYA can be used, but the CYA:FAC ratio should not be greater than 14:1 to keep FAC activity at least as fast as that of chloramine. For example, if at least 3 ppm of FAC is used, then 42 ppm (3 x 14) of CYA would be acceptable.
Using the current EPA restrictions on chlorine concentrations (1 ppm minimum and 4 ppm maximum), the acceptable CYA operating range can be determined.
For a pool where there are large fluctuations in chlorine concentrations and the full range of 1-4 ppm chlorine is needed, the maximum CYA concentration should be 14 ppm to ensure that the 14:1 ratio is never exceeded.
For a pool that is able to maintain closer control and never let the chlorine concentration go below 3 ppm, the aforementioned maximum CYA concentration would be 42 ppm to ensure that the 14:1 ratio is never exceeded.
Following is a more detailed discussion of these topics.
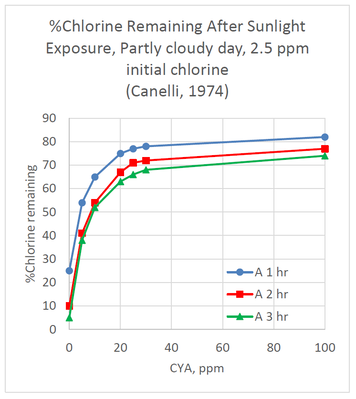
The effect of CYA on chlorine stabilization is well known and is taught in most pool operator training courses. CYA is used to stabilize chlorine from degradation arising from UV radiation in sunlight. The following graph shows the % chlorine remaining after exposure to sunlight for 1 hr, 2 hr and 3 hr periods.
The graph shows that the effect of CYA is not linear, with the greatest improvement in chlorine retention seen with the first
5 ppm of CYA. Without CYA, only 25% of the chlorine remained after one hour of sunlight exposure. Adding only 5 ppm CYA, chlorine retention increased to 54% after one hour. The retention increased to 65% and 75%, after one hour, for 10 ppm and 20 ppm CYA, respectively. Very little additional benefit was seen by increasing CYA concentrations up to 100 ppm for 1 hr, 2 hr, or 3 hr exposure times.
In order to understand the effect of CYA on disinfection, it is important to understand the chemistry of chlorine and CYA.
The amount of HOCl in the water is governed by the pH due to the following equilibrium:
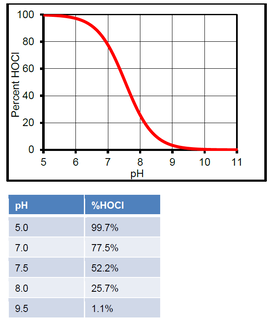
In water, HOCl can split up into hypochlorite ions (OCl-) and hydrogen ions (H+). The amount that it splits up will depend on the pH. At low pH, there will be more HOCl. At high pH, there will be more OCl-. The %HOCl is shown in the following graph and table.
The hypochlorite ion is not nearly as effective in killing pathogens as HOCl. Thus it is advantageous to ensure the pH does not drift above pH 7.8 as it will hinder chlorine efficacy.
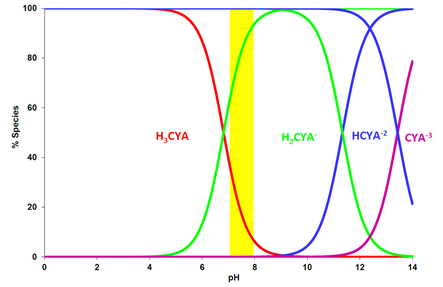
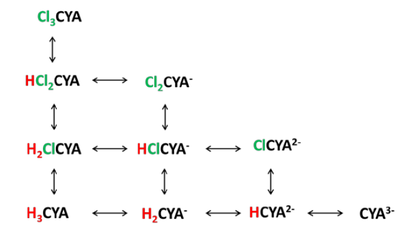
Similar to HOCl, hydrogen ions can split off from the molecule in water and the dissociation is pH dependent. The following graph shows CYA and its three dissociated forms versus pHx.
Since CYA can bind up to three hydrogen ions or three chlorine atoms, the equilibria can be complex. Following is a figure showing these equilibria.
In the late 1960’s and early 1970’s a Harvard graduate student, Joseph O’Brien, measured the equilibria between these species and published equilibrium constants for these reactionsxi. These equilibrium constants can be used to calculate how much of each species is present for given conditions.
Looking at an example of a pool with a FAC of 1 ppm (FAC as measured with a typical pool test kit) and a pH of 7.5, the concentration of HOCl will vary based on the CYA concentration. For a pool with no CYA, the HOCl content is 0.52 ppm, while a pool with 50 ppm CYA would have an HOCl content of 0.01 ppm.
Using these calculations, it is easy to see that less HOCl, the active form of chlorine, is available in the presence of CYA.
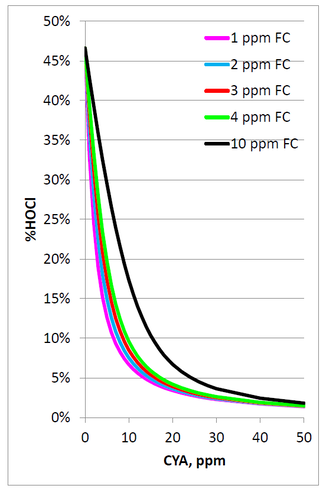
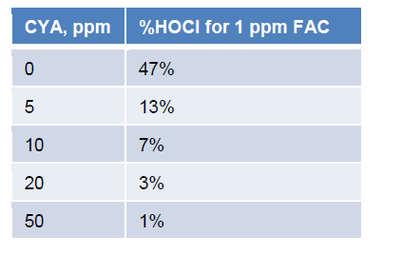
The following graph and table show the %HOCl for various FAC concentrations with pH 7.5, 800 ppm Total Dissolve Solids (TDS), and a temperature of 85°F.
These data clearly show that increasing CYA concentrations will lead to decreasing concentrations of the active form of chlorine, HOCl.
While the practical world does not adhere strictly to this logic, in general, increased levels of a biocide (i.e. something that kills living organisms) will kill things more quickly. If lower levels are used, it will take longer.
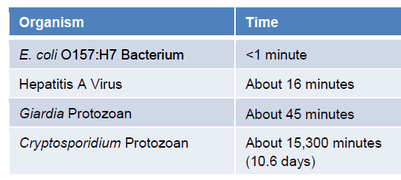
The CDC has posted some typical chlorine CT values on their healthy swimming web site (www.healthyswimming.org). These values are also available in the Annex to CDC’s Model Aquatic Health Code (MAHC). The values are for tests conducted with 1 ppm FAC at pH 7.5, 77°F and no CYA.
This data shows that bacteria like E. coli are fairly easy to kill – 1 ppm FAC can usually kill them in less than a minute. Crypto is an entirely different matter. Even if a pool is kept at 1 ppm free chlorine, crypto can survive for over 10 days.
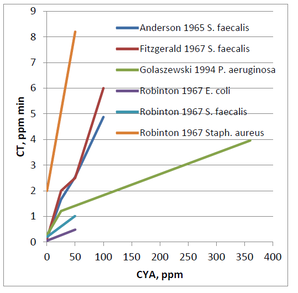
As you might expect from the decreased HOCl concentrations in the presence of CYA, the kill rates of organisms are slower. Following is a graph of CT values summarizing several sets of data on bacteria published in the 60’s
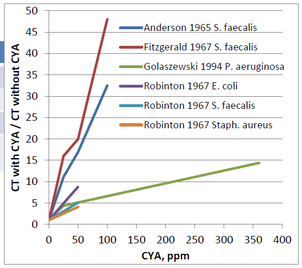
Comparing the ratio of these CT values (CT with CYA/ CT without CYA) vs the CYA allows for a more relative comparison in the change in CT value.
Using this second graph, the impact on the relative increase in CT value is much clearer. For instance, Fitzgerald saw a >45 fold increase in CT value in the presence of 100 ppm CYA.
For all the different researchers looking at the various organisms, it is clear from the first graph the CT values are increasing with increasing CYA concentrations. However, the lines are not all on top of each other. Based on this data, it is impossible to make a general statement such as “50 ppm CYA will always cause a 10-fold increase in CT value.” That might be true for the E. coli data generated by Robinton. However, the Pseudomonas aeruginosa data generated by Golaszewski shows a 5-fold increase, and the Fitzgerald data for Streptococcus faecalis shows a 20-fold increase. Due to the differences in species data, it is challenging to make a definitive statement regarding the allowable concentration of CYA in pools.
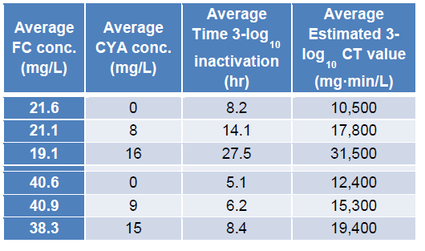
One way to estimate the maximum allowable CYA level is to look at the CDC’s data for crypto. CDC investigated the effect of CYA on kill rates of crypto with chlorine (Murphy 2015) as follows:
Using this data, the CDC established a diarrheal fecal accident response protocolxvi. This protocol specifies that the CYA level must be reduced to 15 ppm to perform the remediation because the CDC was unable to get 3-log reduction of crypto with >16 ppm CYA.
To prevent having to drain at least part of the pool before performing a fecal remediation treatment with chlorine, the CYA level should be kept below 15 ppm. The majority of chlorine stabilization occurs at CYA dosages <15 ppmxvii. Increased CYA concentration has diminishing returns on the chlorine stabilization while increasing the CT values.
Another approach to setting a maximum CYA concentration is to compare the activity of chlorine to that of chloramine. Chloramine is used by municipal drinking water facilities to disinfect water. In this application, there are long residence times in the water distribution system, so a slow acting sanitizer is acceptable. However in a pool where swimmers are in close proximity to each other and fecal-oral transmission of disease has been documentedxviii, a fast acting sanitizer is needed to prevent bather-to-bather disease transmission. Chloramine does not act quickly enough and so has not been accepted by the EPA as a registered sanitizer for pools.
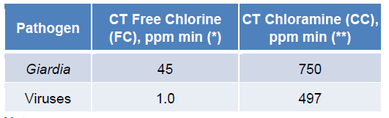
The following table contains CT values from one of EPA’s drinking water treatment guidance manualsxix. As seen in this table, the CT values for monochloramine are much higher than those for free chlorine. For Giardia, chloramine is 16.7 times slower than free chlorine (750/45 = 16.7). For viruses, it is 497 times slower. In a drinking water treatment system, where there is ample time for reaction within the distribution system, these slower kill rates are acceptable. However, in a pool environment, chloramines are not considered sufficient disinfectants.
Notes:
* pH 7.5, 25 °C, 3 log, 1 ppm FC
** pH 6-9, 25 °C, 3 log, 2-10 ppm monochloramine. The 2-10 ppm monochloramine concentration information was obtained from Guidance Manual for Compliance with the Filtration and Disinfection Requirements for Public Water Systems Using Surface Water Sources, EPA 1991.
Giardia is a pathogen that is relevant to both drinking water and pool water. In 1999, samples of formed stools from chlorinated swimming pools were submitted to the CDC for analysisxx. Giardia was found in 4.4% of the samples. Outside of overt fecal accidents, it has been estimated that each swimmer releases an average of 0.14 g of fecal material when swimmingxxi. Giardia outbreaks are regularly reported in the CDC’s outbreak surveillance summaries (Hlavsa 2015).
The EPA data for Giardia inactivation, where chloramine is 16.7 times slower than free chlorine, can be taken as a benchmark to determine the level of sufficient disinfection. In other words, sufficient HOCl should be maintained in the water to ensure that Giardia kill rates with chlorine are no slower than Giardia kill rates with monochloramine.
Unfortunately, kill rates for Giardia are extremely difficult to obtain due to the fact that the only reliable means of obtaining the data requires the use of live mammals for testing the viability of Giardia after it is treated with disinfectant. While no tests have been conducted studying the effect of CYA on the kill rate of Giardia with chlorine, it is still possible to estimate the effect of CYA by looking at the concentration of HOCl at various CYA concentrations.
The idea that pathogen kill rates in the presence of CYA are directly proportional to HOCl concentrations has been proposed by Engel (1983) in his work with Naegleria gruberi xxiii. Gardiner concluded that the data strongly suggests that the bactericidal action of chlorinated cyanurate itself, although present in much larger concentration than HOCl, is negligible. Saita’s 1998 linear plots of survival ratios of poliovirus vs. the molar ratio of CYA : chlorine showed a similar relationshipxxii. Gardiner (1973) also showed that the time required for 99% kill of S. faecalis plotted versus the ratio of CYA : chlorine resulted in a straight linexxiv. The work of Gardiner, Saita and Engel shows that for bacteria, viruses and amoeba, the kill rates in the presence of CYA are proportional to the HOCl concentration, not the total free chlorine concentration.
The following procedure was used to determine the maximum level of CYA that may be used to ensure that the kill rate is no slower than 1 ppm monochloramine (i.e. 16.7 times slower than 1 ppm unstabilized free chlorine). This was done by calculating the maximum level of CYA that will ensure that the HOCl concentration is not decreased more than 16.7 times the concentration without CYA.
The HOCl concentration may be calculated using equilibrium constants published by O’Brien. Using the water chemistry parameters from the EPA table above (pH 7.5, Temperature 25°C, 1 ppm FAC) and a TDS value of 800 ppm, the concentration of HOCl is 0.360 ppm.
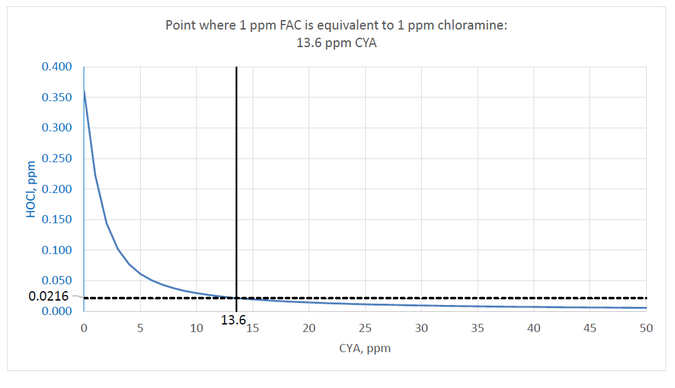
Based on the Giardia data from EPA and to ensure that the kill rate is not any slower than monochloramine, the target should be to maintain an HOCl concentration of at least 0.360/16.7 ppm or 0.0216 ppm.
Following is a chart of values calculated using the following conditions: pH 7.5, TDS 800 ppm, temperature 25°C, 1 ppm FAC. The blue line shows the decrease in HOCl with increasing CYA concentration. The aforementioned point where the HOCl concentration reaches 0.0216 ppm is at 13.6 ppm CYA.
If the minimum chlorine concentration for stabilized and unstabilized pools is 1 ppm, then the limit for CYA should be 13.6 ppm to ensure that chlorine is not less effective than 1 ppm chloramine.
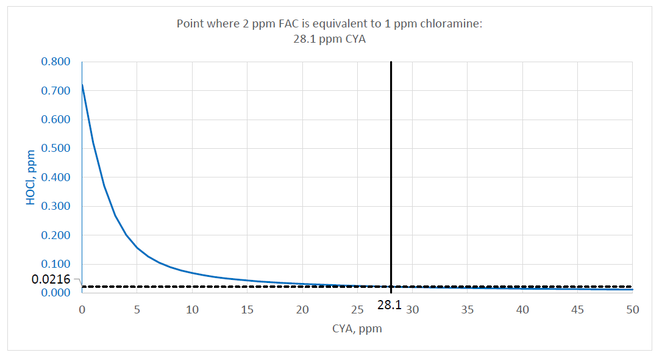
However, the MAHC specifies that the minimum chlorine concentration for stabilized pools is 2 ppm. Recalculating the values using 2 ppm FAC results in the next chart.
The point where the HOCl concentration (blue line) reaches 0.0216 ppm is at 28.1 ppm CYA. Given the 2 ppm FAC minimum in the MAHC, the maximum allowable level of CYA should be 28.1 ppm to ensure that chlorine is not less effective than 1 ppm chloramine.
To maintain the efficacy of FAC as compared to monochloramine, the concentration of CYA should not exceed 14 ppm for every ppm of FAC. If higher levels of chlorine are maintained, then higher levels of CYA can be used, but the CYA:FAC ratio should not be greater than 14:1 to maintain FAC activity at least as fast as that of chloramine. For example, if at least 3 ppm of FAC is used, then 42 ppm (3 x 14) of CYA would be acceptable.
Using the current EPA restrictions on chlorine concentrations (1 ppm minimum and 4 ppm maximum), the acceptable CYA operating range can be determined.
For a pool where there are large fluctuations in chlorine concentrations and the full range of 1-4 ppm chlorine is needed, the maximum CYA concentration should be 14 ppm to ensure that the 14:1 ratio is never exceeded.
For a pool that is able to maintain closer control and never let the chlorine concentration go below 3 ppm, the aforementioned maximum CYA concentration would be 42 ppm to ensure that the 14:1 ratio is never exceeded.
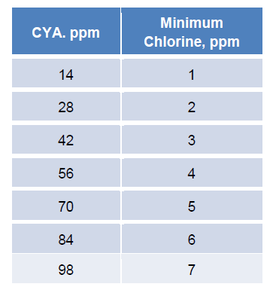
The MAHC allows chlorine concentrations as high as 10 ppm. The following table shows the minimum chlorine concentration that would be required for various CYA concentrations.
Given a CYA concentration of 70 ppm, the minimum chlorine concentration would need to be 5 ppm. Since the CDC’s maximum chlorine concentration is 10 ppm, this would allow the pool to operate with chlorine concentrations anywhere between 5 and 10 ppm. However, operation in this range would be in violation of EPA chlorine labels which do not allow chlorine concentrations higher than 4 ppm in pools and 5 ppm in spas. As such, to maintain pool chlorine concentrations within the EPA mandated range of 1-4 ppm and retain chlorine efficacy, CYA should be maintained at no more than 14 ppm.
While not a public health issue, algae is certainly an issue for pool owners. Accordingly, Lonza has generated data specific for swimming pools to determine the effect of CYA on controlling algae with chlorine.
Eight identical 6,800 gallon pools were operated for three months at a test facility in Miami FL: two control pools with no CYA, two pools at 25-50 ppm CYA, two pools at 100-125 ppm CYA, and two pools at 200-250 ppm CYA. Algae and synthetic bather load were added to the pools once a week. Each week, 2 days after the contaminant additions, the pools were shocked with 10 ppm available chlorine using calcium hypochlorite. The following chart shows that increasing CYA concentrations led to increased algae counts.
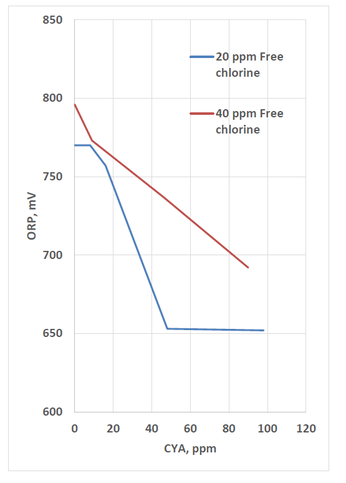
In the CDC’s work with crypto (Murphy 2015), ORP was measured for various chlorine and CYA combinations. The results below show that increasing CYA results in decreasing ORP.
i Murphy, J.L., Arrowood, M. J., Lu, X., Hlavsa, M.C., Beach, M.J., Hill, V.R., 2015, Effect of cyanuric acid on the inactivation of Cryptosporidium parvum under hyperchlorination conditions, Environmental Science and Technology, 49(12), 7348-7355.
ii Model Aquatic Health Code, Centers for Disease Control and Prevention, 2016.
iii G. C. White, Handbook of Chlorination, Second Edition, Van Nostrand Reinhold Company, New York, 1986, page 153.
iv Pool and Spa Operator Handbook, National Swimming Pool Foundation, Colorado Springs Co, 2017.
v O’Brien, J. E. Morris, J. C., Butler, J. N., Equilibria in aqueous solutions of chlorinated isocyanurates, In: Chemical Water Supply Treatment, Chapter 14, pp. 333-358; edited by A. J. Rubin, Ann Arbor Sciences, Ann Arbor, MI 1974. Presented at the District Symposium, Philadelphia, 1973.
vi EPA LT1ESWTR Disinfection Profiling and Benchmarking, 2003 EPA 816-R-03-004
viii Canelli, E.D., Chemical, bacterillogical, and toxicological properties of cyanuric acid and chlorinated isocyanurates as applied to swimming pool disinfection, a review, AJPH February 1974, 64(2) 155-162.
ix Dissociation constant from G. C. White, Handbook of Chlorination, Second Edition, Van Nostrand Reinhold Company, New York, 1986
x O’Brien, J. E. Morris, J. C., Butler, J. N., Equilibria in aqueous solutions of chlorinated isocyanurates, In: Chemical Water Supply Treatment, Chapter 14, pp. 333-358; edited by A. J. Rubin, Ann Arbor Sciences, Ann Arbor, MI 1974. Presented at the District Symposium, Philadelphia, 1973.
xi O’Brien, J. E., Hydrolytic and ionization equilibria of chlorinated isocyanurate in water, Ph.D. Dissertation, Cambridge, MA: Harvard University, 1972.